Does a parallelogram have a line of symmetry

Lines of symmetry in a parallelogram vary from type to type.

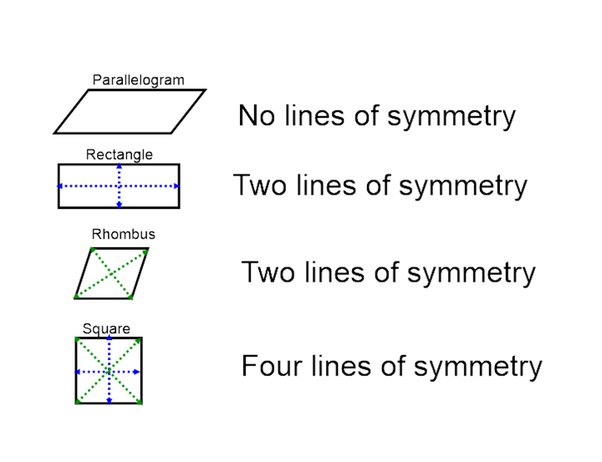
General parallelogram has no lines of symmetry. Some specific types of parallelogram do. See below. Rhombus is a special type of parallelogram and it has two lines of symmetry - its diagonals. Rectangle, which is not a square, has two lines of symmetry - two lines going through the midpoints of opposite sides. Square is a special type of parallelogram with four lines of symmetry - two diagonals and two lines going through the midpoints of opposite sides.

Does a parallelogram have a line of symmetry

In this article, we will study about lines of symmetry in a parallelogram, how to do lines of symmetry, how many lines of symmetry is in a parallelogram. A parallelogram is a type of quadrilateral in which the opposite sides are parallel and equal. A parallelogram is a quadrilateral with opposite sides that are parallel and equal. The line of symmetry is the imaginary line formed as a result of folding a figure to obtain the symmetrical halves. Lines of symmetry in a parallelogram are those that divide a parallelogram into two halves, each of which is the mirror image of the other. We know that parallelograms are classified based on their shapes, line segments, and corners. As a result, they have different symmetry lines and a different number of symmetry lines. By folding a shape and looking for the Line of Symmetry, we can determine whether it is symmetrical. If the folded part fits perfectly on top, with all edges and corners matching, the folded line represents a Line of Symmetry, and the shape is symmetrical along its length, breadth, or diagonals. A line of symmetry is a line that exactly cuts a shape in half. This means that if you folded the shape along the line, both halves would exactly match. Similarly, if you placed a mirror along the line, the shape would not change. A line of symmetry is a mathematical reflection that maps any point on the figure back to the figure.

Students should return to this task both in middle school and in high school to analyze it from a more sophisticated perspective as they develop the tools to do so. Thus, it is important to check whether the lines of symmetry divide the figure not only in equal parts but as mirror images also. This means that if you folded the shape along the line, both halves would exactly match.

Before we begin with the lines of symmetry of a parallelogram, we need to understand the concept of a parallelogram, its properties, its sides, angles and the corresponding relationships. A parallelogram can be defined as a special or unique kind of quadrilateral which is a closed four-sided figure with each of the opposite sides that are parallel to each other and have equal length. The parallelogram has no lines of symmetry and, as with the rectangle, students should experiment with folding a copy to see what happens with the lines through the diagonals as well as horizontal and vertical lines. For understanding the line of symmetry we need to analyse what exactly a line of symmetry is. We can say that a line of symmetry is an axis or imaginary line that can pass through the centre of a shape, facing in any direction, in such a manner that it represents mirror images of each other when cut into two equal halves for example if we cut a square or rectangle, it will have a line of symmetry because at least one imaginary line can be drawn through the centre of the shape that cuts it into two equal halves in such a manner that mirror images of each other are provided. A shape can have multiple lines of symmetry given its properties etc.

Before we begin with the lines of symmetry of a parallelogram, we need to understand the concept of a parallelogram, its properties, its sides, angles and the corresponding relationships. A parallelogram can be defined as a special or unique kind of quadrilateral which is a closed four-sided figure with each of the opposite sides that are parallel to each other and have equal length. The parallelogram has no lines of symmetry and, as with the rectangle, students should experiment with folding a copy to see what happens with the lines through the diagonals as well as horizontal and vertical lines. For understanding the line of symmetry we need to analyse what exactly a line of symmetry is. We can say that a line of symmetry is an axis or imaginary line that can pass through the centre of a shape, facing in any direction, in such a manner that it represents mirror images of each other when cut into two equal halves for example if we cut a square or rectangle, it will have a line of symmetry because at least one imaginary line can be drawn through the centre of the shape that cuts it into two equal halves in such a manner that mirror images of each other are provided. A shape can have multiple lines of symmetry given its properties etc. After looking at the key characteristics and other observations, it turns out that a parallelogram does not have any line of symmetry. It is a very curious question if we ask that why doesn't the parallelogram not have lines of symmetry, will the simplest answer to this question can be that it is impossible to construct a line of symmetry, an axis or an imaginary line that passes through the centre cutting its image in half where each side would represent a mirror image of the other, in order to test this you can simply try and construct a line of symmetry on any parallelogram and figure out that it is almost impossible. After reviewing the characteristics properly and analyzing a parallelogram from all the sides we can conclude that parallelograms do not have any lines of symmetry in turn after reviewing the properties of parallelograms namely that they are quadrilaterals, we can conclude that shapes like squares and rectangles do have lines of symmetry.

Does a parallelogram have a line of symmetry

A parallelogram is a type of quadrilateral where the opposite sides are parallel and equal. The imaginary line so formed along which you can fold a figure to obtain the symmetrical halves is referred to as the line of symmetry. Thus, the lines of symmetry of a parallelogram refer to the lines cutting the parallelogram into two identical parts. Also, the lines of symmetry in a parallelogram vary as per the type of parallelogram. The lines of symmetry in a parallelogram are those lines that divide a parallelogram into two halves such that each half is the mirror image of the other. We know that there are different parallelograms categorized as per their shapes , the line segments, and corners they are made up of. Thus, these have different lines of symmetry and different numbers of symmetry lines. We can find whether a shape is symmetrical by folding it and checking for the Line of Symmetry. If the folded part sits perfectly on top, with all edges and corners matching, then the folded line represents a Line of Symmetry and that shape is symmetrical either along its length, breadth, or diagonals. Let's check for the lines of symmetry in a general parallelogram:.

Lucky number slevin stream english

And, if a parallelogram has line symmetry, what would parallelogram lines of symmetry look like in the form of a diagram. For the square, it can be folded in half over either diagonal, the horizontal segment which cuts the square in half, or the vertical segment which cuts the square in half. Now that you understand the key properties and angle relationships of parallelograms, you are ready to explore the following questions:. Put your understanding of this concept to test by answering a few MCQs. A square has diagonal symmetry lines and bisectors of opposite sides. Therefore you cannot make observations based upon symmetry. About Us. In a rhombus, the lines of symmetry are its diagonals. Task Below are pictures of four quadrilaterals: a square, a rectangle, a trapezoid and a parallelogram. Learn more topics related to Mathematics. They are listed below. For example, a square, a rectangle, and a rhombus all have line symmetry because at least one imaginary line can be drawn through the center of the shape that cuts it into two equal halves that are mirror images of each other. Symmetry is the characteristic of being composed or created of exactly equivalent parts facing each other or around an axis. Rotational Symmetry of a Parallelogram 4. While parallelograms do not have line symmetry, they do have rotational symmetry!

Lines of symmetry in a parallelogram vary from type to type. In simple words, the parallelogram lines of symmetry refer to the lines which cut the parallelogram into two identical parts.

Below are the explanations on the lines of symmetry in each of these parallelograms. Before we begin with the lines of symmetry of a parallelogram, we need to understand the concept of a parallelogram, its properties, its sides, angles and the corresponding relationships. It has 4 lines of symmetry - two diagonals and two lines running through the central points of opposite sides. Explore math program. A parallelogram is a quadrilateral with two pairs of parallel sides, the opposite sides being equal in length and the opposite angles being equal in measure in Euclidean geometry. Put your understanding of this concept to test by answering a few MCQs. The figure below, summariezes why the total number of lines of symmetry in a parallelogram is zero. Cardinal Numbers. Student View. A parallelogram has rotational symmetry since the same figure will occur after the original, or pre-image, has been rotated degrees. In this article, we will study about lines of symmetry in a parallelogram, how to do lines of symmetry, how many lines of symmetry is in a parallelogram. Solution: We know that a rhombus has 4 equal sides but what about diagonals? Thus, these have different lines of symmetry and different numbers of symmetry lines. A parallelogram is a quadrilateral whose opposite sides are equal and parallel. Which parallelogram contains the most symmetry lines?