Factors of 240 in pairs

Being an even number, it is a multiple of various numbers like 2, 3, 5 and 10, hence it has many factors. We can say, is a refactorable number, because it is divisible by the count of its divisors.

Because has so many factors, it is possible to make MANY different factor trees that create a forest of factor trees. This post only contains eleven of those many possibilities. The two trees below demonstrate different permutations that can be made from the same basic tree. The mirror images of both, as well as mirror images of parts of either tree, would be other permutations. A good way to make a factor tree for a composite number is to begin with one of its factor pairs and then make factor trees for the composite numbers in that factor pair.

Factors of 240 in pairs

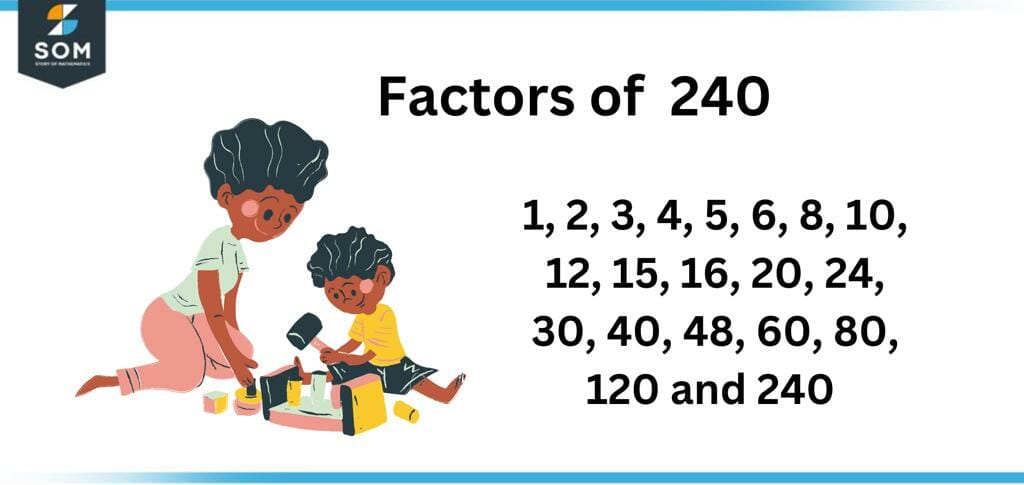
A factor of is a number that divides exactly, that is, without any remainder. The factors of cannot be a fra ction or a decimal. In the following article, we will be able to learn about the factors of and will also be able to understand how we can find the factors of Read More Read Less. The numbers that divide without leaving any remainder are known as the factors of For example, 6 is a factor of because when we divide by 6, it gives us 40 as the quotient, and 0 as the remainder. Here, the quotient is also a factor of So, to check if a number is a factor of or not, divide by that number and check if the remainder is zero or not. The following table shows us how we can obtain the factors of by applying divisibility rules and division facts. The factors of are 1, 2, 3, 4, 5, 6, 8, 10, 12, 15, 16, 20, 24, 30, 40, 48, 60, 80, and The number is a composite number, that is, it has more than two factors. To find the prime factors of , first we will divide by its smallest prime factor, that is, 2. Now, divide by the next prime number, that is, 3, as 15 is not divisible by 2. Now, divide by the next prime number, that is, 5, as 5 is not divisible by 3.

View Test Series.

Factorisation provides us with the factors of any number. It defines the process of determining the factors of a number in a systematic approach so that all of the factors are noticed. A factor of any given integer may be defined as the divisor that divides it completely, leaving no remainder behind. We can employ any of the techniques among the division method, the factorisation method, or the prime factorisation method to identify the factors of any integer. The factors can be positive integers or negative integers.

A factor of is a number that divides exactly, that is, without any remainder. The factors of cannot be a fra ction or a decimal. In the following article, we will be able to learn about the factors of and will also be able to understand how we can find the factors of Read More Read Less. The numbers that divide without leaving any remainder are known as the factors of

Factors of 240 in pairs

The factors of are the numbers that divide exactly without leaving any remainder. The factors and the pair factors of can be expressed in positive or negative forms. For example, the pair factor of can be written as 1, or -1, If we multiply a pair of negative numbers, such as multiplying -1 and results in the original number.

French actress brigitte bardot

The factorization method splits the referred number into all the possible operations of multiplication, each involving the factor pair of any two integers, such that the obtained product is always the original number whose factors are sought. Positive factor pairs and negative factor pairs are the two types of factor pairs. The prime factors are multiplied together to obtain the original number. So, it is evident that both a and b are the factors of the number X. All of these factors can be used to divide by and get a whole number. So the way you find and list all of the factors of is to go through every number up to and including and check which numbers result in an even quotient which means no decimal place. Step: 4 The numbers 15 and 16 can now both be factored further because they are composite numbers. Similarly, the negative factors of are -1, -2, -3, -4, -5, -6, -8, , , , , , , , , , , , and Determinants And Matrices. If the integer divides exactly without leaving a remainder, then the integers are the factors of Now, divide the quotient by the smallest but divisible prime number and write below it. So, the smallest factor of is 1, and the greatest factor is itself. Divide the given integer by the smallest possible prime number that completely divides the provided integer. What are the Factors of ?

Being an even number, it is a multiple of various numbers like 2, 3, 5 and 10, hence it has many factors. We can say, is a refactorable number, because it is divisible by the count of its divisors. In this mini lesson let us learn to calculate all the factors of , the factors of in pairs and the prime factorization of

Again, divide the number 60 by the lowest divisible prime number 2 to get the quotient Factors of Solved Examples Example 1: Sandra decides to travel to her native at miles. Whenever an integer is multiplied by another integer, the resultant product, which is also an integer, is the multiple of the first and the second integers. So, it is inferred that the number Y is the multiple of both the numbers b and c. Factoring Algebraic Expressions. Just make sure to pick small numbers! Whenever an integer divides any number completely, leaving no remainder, the referred integer is the factor of that number. Place Value Worksheets Grade 5. Next, divide the number by the lowest divisible prime number 2 to get the quotient of A shared or joint factor between two or more numbers is known as a common factor. The factor tree of can be represented as: This means that 2, 3 and 5 are the prime factors of Solved Examples of Factors of Example: 1 Whta to numbers multiply to Factors of that add up to 8. What Are The Factors of ?