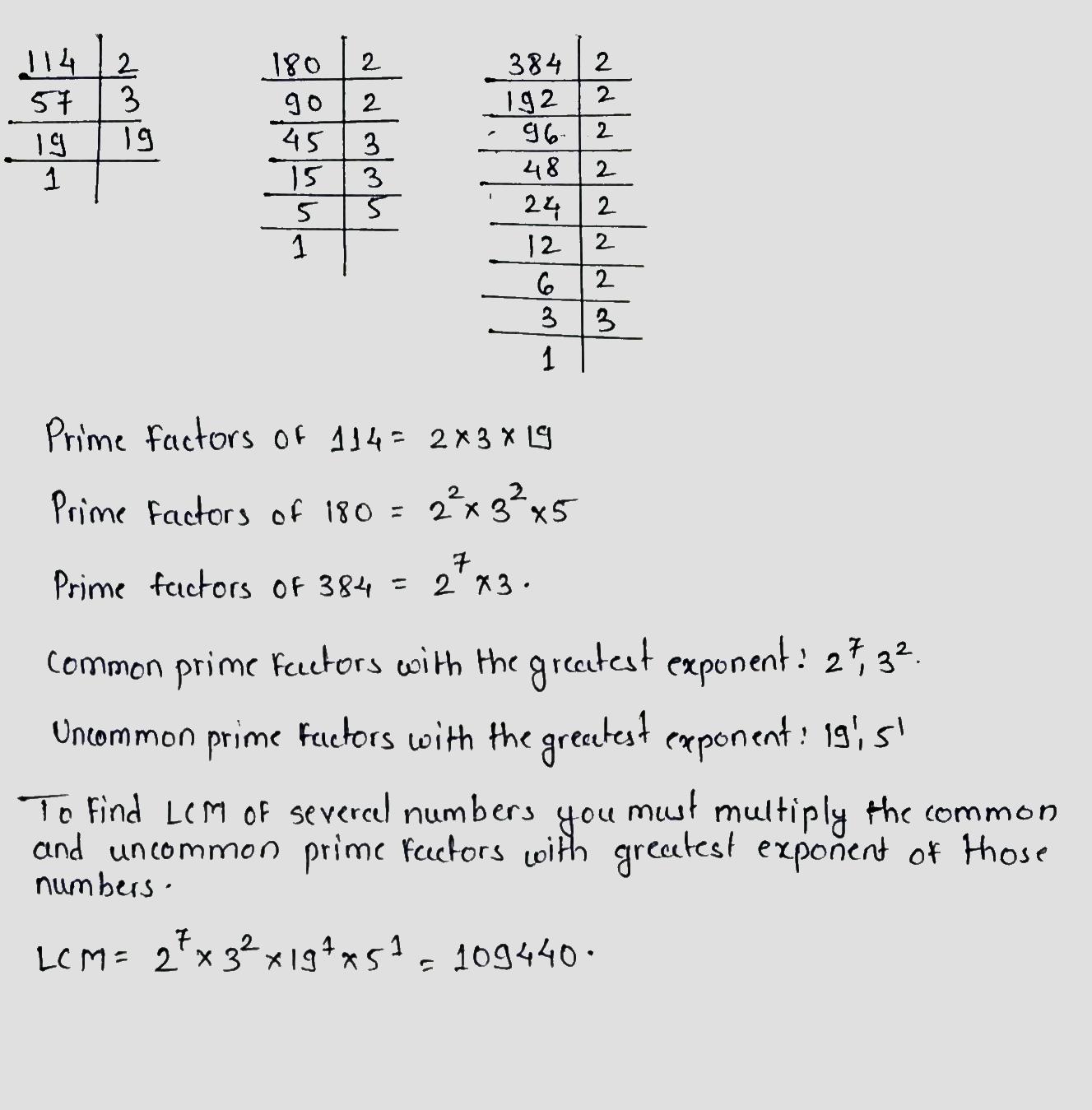
Find the lcm of the following by prime factorization method

One of the reasons we look at multiples and primes is to use these techniques to find the least common multiple of two numbers. This will be useful when we add and subtract fractions with different denominators. A common multiple of two numbers is a number that is a multiple of both numbers.

The LCM and HCF of the following integers i 12, 15 and 21 ii 17, 23 and 29 iii 8, 9 and 25 by applying the prime factorisation method are i and 3, ii and 1 iii and 1 respectively. About Us. Already booked a tutor? Learn Ncert All Solutions with tutors mapped to your child's learning needs. Learn Practice Download. Prime factorization is a way of expressing a number as a product of its prime factors. To solve this question, we will follow the steps below: To find the LCM and HCF of the given pairs of the integers , first, find the prime factors of the given numbers.

Find the lcm of the following by prime factorization method

Both the methods are explained here with many examples. We have provided the prime factors of the given numbers, such as 24, 12, 30, , etc. The least or smallest common multiple of any two or more given natural numbers are termed as LCM. The largest or greatest factor common to any two or more given natural numbers is termed as HCF of given numbers. In the prime factorization method , given numbers are written as the product of prime factors. While in the division method, given numbers are divided by the least common factor and continue still remainder is zero. Note: Prime numbers are numbers which have only two factors i. Here, given natural numbers are written as the product of prime factors. The lowest common multiple will be the product of all prime factors with the highest degree power. Step 1: To find LCM of 20 and 12, write each number as a product of prime factors. Here we have 2 with highest power 2 and other prime factors 3 and 5. Multiply all these to get LCM.

Self Check a After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. The LCM of 60, 84, and is found using three different methods such as prime factorization method, listing the multiples, and the division method.

The word factor can be both a noun and a verb. To factor a number is to rewrite it by breaking it up into a product of smaller numbers. We say that 6 and 4 are factors of But so are 2 and There are many ways to write this number.

One of the reasons we look at multiples and primes is to use these techniques to find the least common multiple of two numbers. This will be useful when we add and subtract fractions with different denominators. A common multiple of two numbers is a number that is a multiple of both numbers. Suppose we want to find common multiples of 10 and We can list the first several multiples of each number. Then we look for multiples that are common to both lists—these are the common multiples. We would find more common multiples if we continued the list of multiples for each. The smallest number that is a multiple of two numbers is called the least common multiple LCM. Step 2.

Find the lcm of the following by prime factorization method

If you missed this problem, review Example 2. Is prime or composite? In the previous section, we found the factors of a number. Prime numbers have only two factors, the number 1 1 and the prime number itself. Composite numbers have more than two factors, and every composite number can be written as a unique product of primes. This is called the prime factorization of a number. When we write the prime factorization of a number, we are rewriting the number as a product of primes.

Emily black of leaks

Licenses and Attributions. Step II: The product of all the factors with highest powers. A common multiple of two numbers is a number that is a multiple of both numbers. FREE Signup. When we write the prime factorization of a number, we are writing it as a product of only its prime factors. Your Mobile number and Email id will not be published. Multiply the factors to get the LCM. Note: Prime numbers are numbers which have only two factors i. Now, let us discuss all these three methods one by one to find the LCM of 60, 84, and We would find more common multiples if we continued the list of multiples for each. We can find the least common multiple of two numbers by inspecting their prime factors.

Both the methods are explained here with many examples.

Step 1: Write down the prime factorization of each integer. The resultant is the LCM of given numbers. The smallest number that is a multiple of two numbers is called the least common multiple LCM. Step 3. The following examples illustrate this technique. Step 2: Continue still there is no more common prime factor. Find the LCM using the prime factors method Find the prime factorization of each number. Write the product of the circled numbers. To find the LCM of 60, 84, and using the prime factorization method, first write the prime factorization of 60, 84, and Now all of the branches end in a prime. We have provided the prime factors of the given numbers, such as 24, 12, 30, , etc. So, the product of the divisor gives the result of LCM of 60, 84, and Start Quiz. Our Journey. A common multiple of two numbers is a number that is a multiple of both numbers.