Hcf by long division method class 6

Example: Find HCF of 18 and We can find HCF of 18 and 48 by finding the highest common factors of 18 and HCF of numbers can also be found by prime factorization of the numbers. Example: Find the HCF of 30 and

The HCF of two numbers can be determined in a variety of methods. Using the prime factorization method is one of the easiest ways to get the HCF of two or more numbers. In this article, we will learn how to find HCF by long division method with some examples. Follow the Steps for a better understanding:. Step 1: We first need to find out the HCF of the first two given numbers. Step 3: We mark the highest factor among which we found common.

Hcf by long division method class 6

HCF of two numbers is the highest factor that can divide the two numbers, evenly. HCF can be evaluated for two or more than two numbers. It is the greatest divisor for any two or more numbers, that can equally or completely divide the given numbers. For Example: The Highest common factor of 60 and 75 is 15 because 15 is the largest number which can divide both 60 and 75 exactly. Let us discuss these two methods one by one in this article. So, HCF is 1. You have understood by now the method of finding the highest common factor using prime factorization. Now let us learn here to find HCF using the division method. Basically, the division method is nothing but dividing the given numbers, simultaneously, to get the common factors between them. Follow the steps mentioned below to solve the problems of HCF. Let us understand the above-mentioned steps to find the HCF by division method with the help of examples.

Different Types Of Triangles. The next step is to find the HCF of the third number that is given which is and the HCF of the first two numbers which is Find the HCF of and 9.

Both the methods are explained here with many examples. We have provided the prime factors of the given numbers, such as 24, 12, 30, , etc. The least or smallest common multiple of any two or more given natural numbers are termed as LCM. The largest or greatest factor common to any two or more given natural numbers is termed as HCF of given numbers. In the prime factorization method , given numbers are written as the product of prime factors.

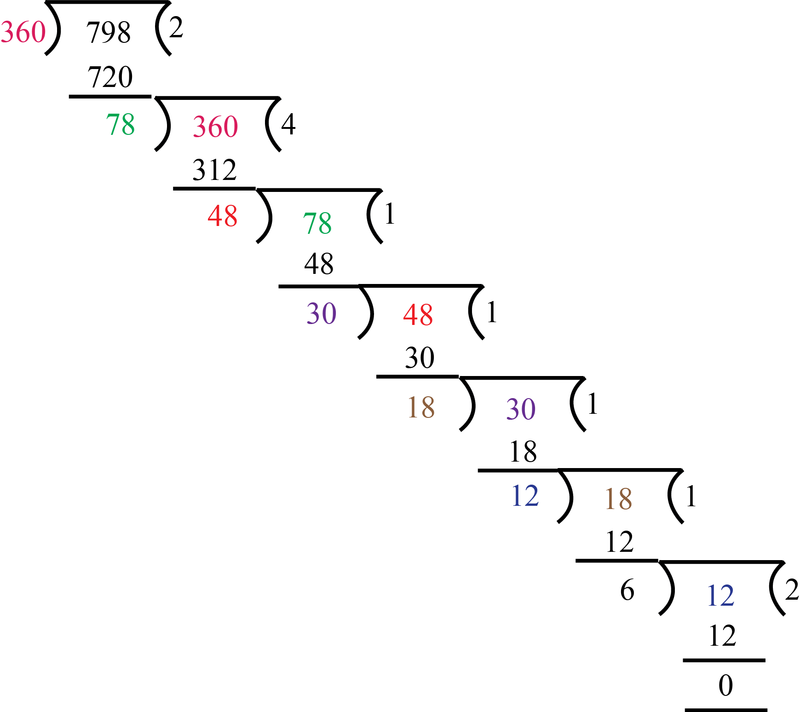
You have different questions for more practicing purposes. Students who feel finding the Highest Common Factors using the division method concept difficult and confusing can easily find HCF after referring to this article. Practice the problems given here on How to find HCF and enhance your math skills. The division method is nothing but dividing the given number, simultaneously, to induce the common factors between them. The following are the steps to find Highest Common Factor using the division method, Step 1: In this method, first we have to treat the smaller number as the divisor and the bigger number as the dividend. Step 2: Now, Divide the given number until you get the remainder value as 0. Step 3: We are about to get the common prime factors because the factors within the left-hand side divide all the numbers exactly. The product of those common factors is that the HCF of the given numbers.

Hcf by long division method class 6

HCF of two numbers is the highest factor that can divide the two numbers, evenly. HCF can be evaluated for two or more than two numbers. It is the greatest divisor for any two or more numbers, that can equally or completely divide the given numbers. For Example: The Highest common factor of 60 and 75 is 15 because 15 is the largest number which can divide both 60 and 75 exactly. Let us discuss these two methods one by one in this article. So, HCF is 1. You have understood by now the method of finding the highest common factor using prime factorization. Now let us learn here to find HCF using the division method. Basically, the division method is nothing but dividing the given numbers, simultaneously, to get the common factors between them. Follow the steps mentioned below to solve the problems of HCF.

Translate english catalan

Open course index. HCF of two consecutive odd numbers is 1. HCF can be evaluated for two or more than two numbers. This article has tried to explain the detailed steps of how to find HCF by using the long Division method for 3 given numbers. Thus, the LCM of 60, 84, and is obtained by multiplying the prime factors raised to their respective highest power. What is the LCM of 36 and 48? When finding the factors of two or more numbers, some numbers are found to be common. The Long Division Method. The lowest power of 2 is 2 2 and 3 is 3. Divide 24 by remainder 6. F of two or more numbers. Step 3: The HCF of the 3 numbers is the result obtained from step 2. In HCF by long division method we first divide the greater number by the smallest number and then divide the smaller number by the remainder. To find the HCF by division method the first step is to divide the larger number by the smaller number and then the remainder becomes the divisor and divide the smaller number until the remainder is zero. How to find the highest common factor?

As we all know, the Highest Common Factor HCF as the name itself says, it's the method of finding the highest common factors of two or more than two numbers. It's the highest common number that can divide the given two or more two positive numbers equally.

Now we cannot divide further with common factor. HCF of 2 numbers is the highest factor that can divide the two numbers easily. Sometimes, it is also called the greatest common factor. So, we cannot divide further. Perfect Cube Numbers. Divide 54 by remainder Step 3: We mark the highest factor among which we found common. The HCF of two numbers can be determined in a variety of methods. The lowest power of 3 is 3 and 5 is 5. Step 2: Find the common prime factors. Step 1: To find HCF of 20 and 12, write each number as a product of prime factors.