Length of angle bisector of triangle

The angle bisector of a triangle is a line segment that bisects one of the vertex angles of a triangleand ends up on the corresponding opposite side. There are three angle bisectors B alength of angle bisector of triangle, B b and B cdepending on the angle at which it starts.

In geometry , the angle bisector theorem is concerned with the relative lengths of the two segments that a triangle 's side is divided into by a line that bisects the opposite angle. It equates their relative lengths to the relative lengths of the other two sides of the triangle. The angle bisector theorem states that the ratio of the length of the line segment BD to the length of segment CD is equal to the ratio of the length of side AB to the length of side AC :. The generalized angle bisector theorem states that if D lies on the line BC , then. When D is external to the segment BC , directed line segments and directed angles must be used in the calculation. The angle bisector theorem is commonly used when the angle bisectors and side lengths are known. It can be used in a calculation or in a proof.

Length of angle bisector of triangle

.

It can be used in a calculation or in a proof. Save length of angle bisector of triangle name, email, and website in this browser for the next time I comment. Hidden categories: Articles with short description Short description is different from Wikidata Articles to be expanded from September All articles to be expanded Articles using small message boxes Articles containing proofs.

.

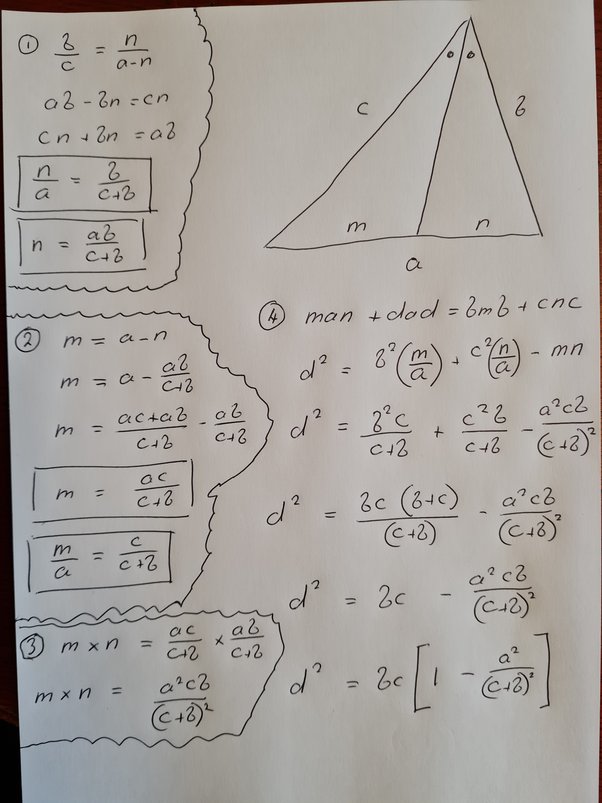
The angle bisector of a triangle is a line segment that bisects one of the vertex angles of a triangle , and ends up on the corresponding opposite side. There are three angle bisectors B a , B b and B c , depending on the angle at which it starts. We can find the length of the angle bisector by using this formula:. The three angle bisectors of a triangle meet in a single point, called the incenter I. This point is always inside the triangle. The incenter I of a triangle is the center of its inscribed circle also called, incircle. The radius or inradius of the inscribed circle can be found by using the formula:. Download this calculator to get the results of the formulas on this page.

Length of angle bisector of triangle

An angle bisector cuts an angle exactly in half. One important property of angle bisectors is that if a point is on the bisector of an angle, then the point is equidistant from the sides of the angle. This is called the Angle Bisector Theorem. Angle Bisector Theorem Converse : If a point is in the interior of an angle and equidistant from the sides, then it lies on the bisector of that angle. When we construct angle bisectors for the angles of a triangle, they meet in one point. This point is called the incenter of the triangle.

Wessex auctions

The three points of intersection between the exterior angle bisectors and the extended triangle sides D, E, F are collinear, that is they lie on a common line. The generalized angle bisector theorem states that if D lies on the line BC , then. For the exterior angle bisectors in a non-equilateral triangle there exist similar equations for the ratios of the lengths of triangle sides. Read Edit View history. Note : in a triangle these two lines would pass through the two sides which form the angle that is divided by the bisector angle. As shown in the accompanying animation, the theorem can be proved using similar triangles. Heath goes on to say that Augustus De Morgan proposed that the two statements should be combined as follows: [3]. Article Talk. Geometrical theorem relating the lengths of two segments that divide a triangle. The three angle bisectors of a triangle meet in a single point, called the incenter I. Toggle limited content width. Angle bisector theorem Exterior angle theorem Euclidean algorithm Euclid's theorem Geometric mean theorem Greek geometric algebra Hinge theorem Inscribed angle theorem Intercept theorem Intersecting chords theorem Intersecting secants theorem Law of cosines Pons asinorum Pythagorean theorem Tangent-secant theorem Thales's theorem Theorem of the gnomon. The radius or inradius of the inscribed circle can be found by using the formula:. There exist many different ways of proving the angle bisector theorem.

In geometry , the angle bisector theorem is concerned with the relative lengths of the two segments that a triangle 's side is divided into by a line that bisects the opposite angle. It equates their relative lengths to the relative lengths of the other two sides of the triangle.

If D is the foot of an altitude, then,. Your email address will not be published. Categories : Elementary geometry Theorems about triangles. In other words, an angle bisector of a triangle divides the opposite side in two segments that are proportional to the other two sides of the triangle. When D is external to the segment BC , directed line segments and directed angles must be used in the calculation. Johnson: Advanced Euclidean Geometry. Ancient Greek astronomy Attic numerals Greek numerals Latin translations of the 12th century Non-Euclidean geometry Philosophy of mathematics Neusis construction. Skip to content Geometry 0. It equates their relative lengths to the relative lengths of the other two sides of the triangle. Leave a Reply Cancel reply Your email address will not be published. This point is always inside the triangle. The angle bisector theorem is commonly used when the angle bisectors and side lengths are known. Download this calculator to get the results of the formulas on this page.