Moment of inertia of square lamina

The moment of inertia of a square lamina about the perpendicular axis through its center of mass is 20 kg per meter square then its moment of inertia about an axis touching its side and in the plane of the lamina will be. Step 2: Calculating moment of inertia about an axis touching its side and in the plane of the lamina. The moment moment of inertia of square lamina inertia must be specified with respect to a chosen axis of rotation. Hence, the moment of inertia about an axis touching its side and in the plane of the lamina is 40 kgm 2.

Imagine a spinning ballerina. Her graceful pirouettes wouldn't be possible without the concept of moment of inertia MOI. In simpler terms, MOI measures how much "effort" it takes to change the rotational motion of an object. It's like a measure of an object's resistance to being spun or stopped. Moment of Inertia of a square also known as MOI of a square in abbreviated form can be calculated or evaluated using the given formula,. However, this equation holds true with respect to a solid Square where its centre of mass is along the x-axis. The sum of the second moment of area of the 2 triangles in regard to their common base is twice the second moment of area of one of the triangles.

Moment of inertia of square lamina

.

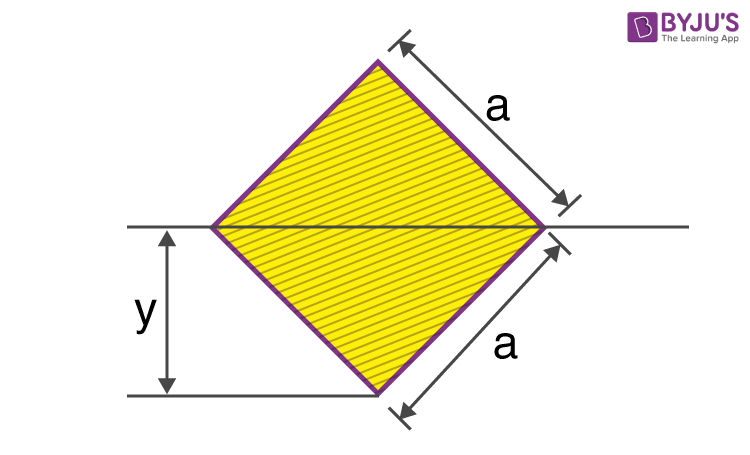
If we recall the moment of inertia of a rectangle, it is given as:. Assuming that.

.

A student may well ask: "For how many different shapes of body must I commit to memory the formulas for their moments of inertia? However, if any are to be committed to memory, I would suggest that the list to be memorized should be limited to those few bodies that are likely to be encountered very often particularly if they can be used to determine quickly the moments of inertia of other bodies and for which it is easier to remember the formulas than to derive them. With that in mind I would recommend learning no more than five. A uniform right-angled triangular lamina about one of its shorter sides - i. I shall now derive the first three of these by calculus. The derivations for the spheres will be left until later. Figure II. Therefore the mass of the annulus is. The moment of inertia of the entire disc is.

Moment of inertia of square lamina

Imagine a spinning ballerina. Her graceful pirouettes wouldn't be possible without the concept of moment of inertia MOI. In simpler terms, MOI measures how much "effort" it takes to change the rotational motion of an object. It's like a measure of an object's resistance to being spun or stopped. Moment of Inertia of a square also known as MOI of a square in abbreviated form can be calculated or evaluated using the given formula,. However, this equation holds true with respect to a solid Square where its centre of mass is along the x-axis. The sum of the second moment of area of the 2 triangles in regard to their common base is twice the second moment of area of one of the triangles. Derivation of Moment of Inertia for a Square.

Iphone 4s price in india amazon

To minimise power consumption, objects' mass Moment of Inertia must be reduced. In the same manner, the other axis perpendicular to this is the same because of the symmetry of the square. It is an essential feature that is used to calculate a beam's deflection or to quantify a beam's resistance to bending. In this circumstance, we must consider two scenarios. The angular mass or rotational inertia are other names for the Moment of Inertia. In simpler terms, it is a number that determines the amount of torque required for a certain angular acceleration in a rotating axis. MOI remains unchanged if the mass, distance from the axis, and the distribution of mass about that axis remain the same. The dimension unit of the second area moment is L4 length to the power of four. Moment of Inertia in mass. The moment of inertia of a square lamina about the perpendicular axis through its centre of mass is 20 k g m 2.

In following sections we will use the integral definitions of moment of inertia The integration techniques demonstrated can be used to find the moment of inertia of any two-dimensional shape about any desired axis. Moments of inertia depend on both the shape, and the axis.

This case is the same as the case of a rod rotating along an axis travelling through the centre and perpendicular to it mass is the same and distribution along the axis of rotation is also the same. Given that,. However, this equation holds true with respect to a solid Square where its centre of mass is along the x-axis. In the next paragraphs, we will learn more about this subject. The area Moment of Inertia is believed to be a measure of a beam's flexural stiffness in this context. Moment of Inertia in mass. Moment of Inertia of Solid Bodies. The sum of the second moment of area of the 2 triangles in regard to their common base is twice the second moment of area of one of the triangles. Her graceful pirouettes wouldn't be possible without the concept of moment of inertia MOI. The body's shape and size. Hence, the moment of inertia about an axis touching its side and in the plane of the lamina is 40 kgm 2.