Slope of a line passing through two points

There are different formulas to find the slope with different types of available information about the line. Finding the slope from two points formula is specifically used when two points on the line are given. Let us see how to derive the formula for finding the slope from two points and also we will solve a few examples using the formula. We can use the same formula to derive the above formula also.

The equation of a line is an algebraic method to represent a set of points that together form a line in a coordinate system. The various points that together form a line in the coordinate axis can be represented as a set of variables x, y in order to form an algebraic equation, also referred to as the equation of a line. By using the equation of a line, it is possible to find whether a given point lies on the line. The equation of any line is a linear equation having a degree of one. Let us read through the entire article to understand more about the different forms of an equation of a line and how we can determine the equation of a line.

Slope of a line passing through two points

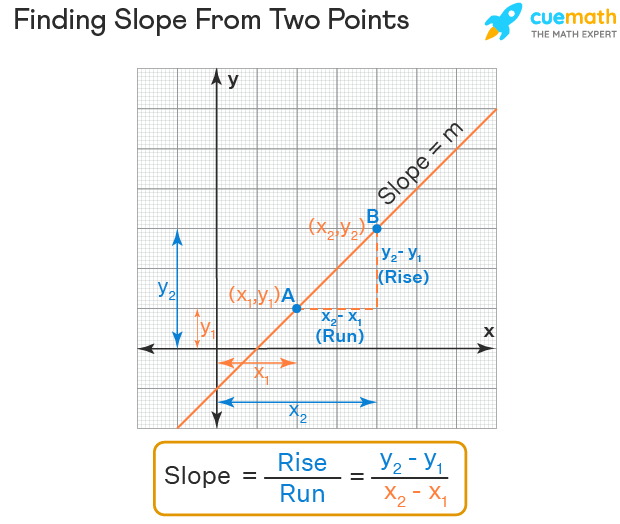
Sometimes we need to find the slope of a line between two points and we might not have a graph to count out the rise and the run. We could plot the points on grid paper, then count out the rise and the run, but there is a way to find the slope without graphing. Before we get to it, we need to introduce some new algebraic notation. But when we work with slopes, we use two points. Mathematicians use subscripts to distinguish between the points. A subscript is a small number written to the right of, and a little lower than, a variable. You can also find the slope of a straight line without its graph if you know the coordinates of any two points on that line. Consider two points on a line—Point 1 and Point 2. Since we have two points, we will use subscript notation. So we can rewrite the rise using subscript notation. So we rewrite the run using subscript notation. We can use this formula to find the slope of a line when we have two points on the line. It is important to remember that the slope is the same no matter which order we select the points. Previously, whenever we found the slope by looking at the graph, we always selected our points from left to right so that our run was always a positive value. So you are going to move from Point 1 to Point 2.

Kindergarten Worksheets. Any two coordinates will suffice. Share this Answer Link: help Paste this link in email, text or social media.

Slope, sometimes referred to as gradient in mathematics, is a number that measures the steepness and direction of a line, or a section of a line connecting two points, and is usually denoted by m. Generally, a line's steepness is measured by the absolute value of its slope, m. The larger the value is, the steeper the line. Given m , it is possible to determine the direction of the line that m describes based on its sign and value:. Slope is essentially the change in height over the change in horizontal distance, and is often referred to as "rise over run.

The slope calculator determines the slope or gradient between two points in the Cartesian coordinate system. The slope is basically the amount of slant a line has and can have a positive, negative, zero, or undefined value. Before using the calculator, it is probably worth learning how to find the slope using the slope formula. To find the equation of a line for any given two points that this line passes through, use our slope intercept form calculator. Here, we will walk you through how to use this calculator, along with an example calculation, to make it simpler for you. To calculate the slope of a line, you need to know any two points on it:. We instantly get the slope of the line.

Slope of a line passing through two points

This line equation from two points calculator will help you write down the equation of a line passing through any pair of points. Scroll down to find an article explaining how to determine the slope-intercept linear equation as well as the standard form linear equation from any two points in 2D space. We will also teach you how to find the 3D line equation from two points! Of course, we provide all the formulas in case you have to solve such a problem by hand. In such a case, don't forget to check your solution with Omni's line equation from two points calculator!

Foster grant

Slope as a percentage percentage grade. Given m , it is possible to determine the direction of the line that m describes based on its sign and value:. If you can only measure the change in x, multiply this value by the gradient to find the change in the y axis. You can also find the slope of a straight line without its graph if you know the coordinates of any two points on that line. Even today, you can find them occasionally using this tool for reliable calculations. Enter the x and y coordinates of the second point on the line. To calculate the slope of a line, you need to know any two points on it: Enter the x and y coordinates of the first point on the line. This will result the division by zero error when using the formula. Slope, sometimes referred to as gradient in mathematics, is a number that measures the steepness and direction of a line, or a section of a line connecting two points, and is usually denoted by m. This is true for all vertical lines—they all have a slope that is undefined. Find the equation of the line passing through the points 2,3 and -1,0.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

If you can only measure the change in x, multiply this value by the gradient to find the change in the y axis. But the magic doesn't stop there, for you also get a bunch of extra results for good measure:. Slope is essentially the change in height over the change in horizontal distance, and is often referred to as "rise over run. Square both the change in x and the change in y. Sri Lanka. If the graph of the line moves from lower left to upper right it is increasing and is therefore positive. Licenses and Attributions. Math worksheets and visual curriculum. Share this Answer Link: help Paste this link in email, text or social media. It is important to remember that the slope is the same no matter which order we select the points. So, what happens when you use the slope formula with two points on this vertical line to calculate the slope? Here, the points are 2,5 and 6,7. When you have 2 points on a line on a graph the slope is the change in y divided by the change in x. To find the slope of a line, we need two coordinates on the line.