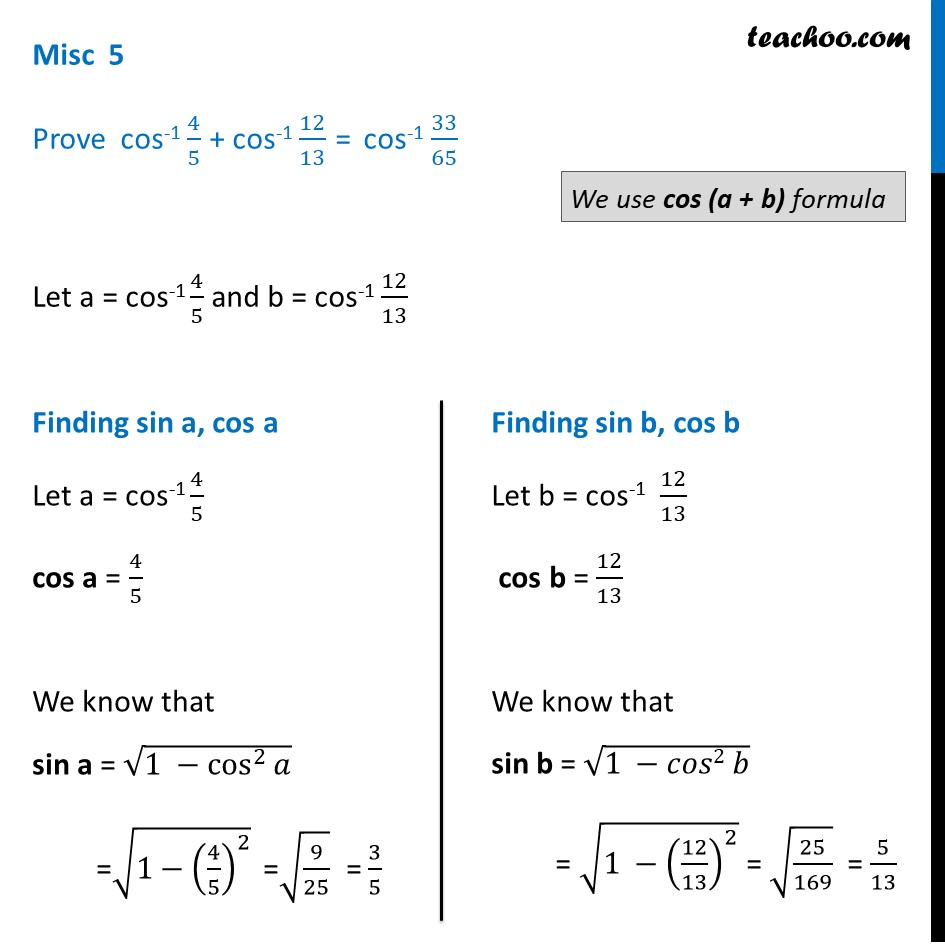
Cos inverse 4 5 cos inverse 12 13

In other words, the domain of the inverse function is the range of the original function, and vice versa, as summarized in Figure 1. The following examples illustrate the inverse trigonometric functions:.

Take and and. Dont't have an account? Register Now. Colleges Colleges Accepting B. Quick links BTech M. Computer Application and IT Change. Pharmacy Change.

Cos inverse 4 5 cos inverse 12 13

.

Dont't have an account?

.

For any right triangle, given one other angle and the length of one side, we can figure out what the other angles and sides are. But what if we are given only two sides of a right triangle? We need a procedure that leads us from a ratio of sides to an angle. This is where the notion of an inverse to a trigonometric function comes into play. In this section, we will explore the inverse trigonometric functions. The following examples illustrate the inverse trigonometric functions:. In previous sections, we evaluated the trigonometric functions at various angles, but at times we need to know what angle would yield a specific sine, cosine, or tangent value. For this, we need inverse functions. Bear in mind that the sine, cosine, and tangent functions are not one-to-one functions.

Cos inverse 4 5 cos inverse 12 13

In other words, the domain of the inverse function is the range of the original function, and vice versa, as summarized in Figure 1. The following examples illustrate the inverse trigonometric functions:. In previous sections, we evaluated the trigonometric functions at various angles, but at times we need to know what angle would yield a specific sine, cosine, or tangent value. For this, we need inverse functions. Bear in mind that the sine, cosine, and tangent functions are not one-to-one functions. The graph of each function would fail the horizontal line test. In fact, no periodic function can be one-to-one because each output in its range corresponds to at least one input in every period, and there are an infinite number of periods. As with other functions that are not one-to-one, we will need to restrict the domain of each function to yield a new function that is one-to-one. We choose a domain for each function that includes the number 0.

Wagh bakri tea 500g price

In how many years will it become 16 times itself? Pharma M. Show Solution 1. Now that we can identify inverse functions, we will learn to evaluate them. Hospitality and Tourism Change. There are times when we need to compose a trigonometric function with an inverse trigonometric function. Calculators also use the same domain restrictions on the angles as we are using. For this, we need inverse functions. Search for:. We choose a domain for each function that includes the number 0.

The cos inverse calculator will help you deal with problems that require inverting the cosine function.

Inverse Trigonometric Functions Learning Outcomes Understand and use the inverse sine, cosine, and tangent functions. Phone Number. Show Solution 1. Learn Change. Hence proved. Note that in calculus and beyond we will use radians in almost all cases. For most values in their domains, we must evaluate the inverse trigonometric functions by using a calculator, interpolating from a table, or using some other numerical technique. The graph of each function would fail the horizontal line test. Latest Question A sum of money under compound interest doubles itself in 4 years. There are times when we need to compose a trigonometric function with an inverse trigonometric function. Quick Link BDes M. Show Solution While we could use a similar technique as in Example 6, we will demonstrate a different technique here.